Geometry is beautiful
We study geometric structures on low-dimensional manifolds, such as hyperbolic or anti-de Sitter structures, representations of surface groups in Lie groups, and their moduli spaces.
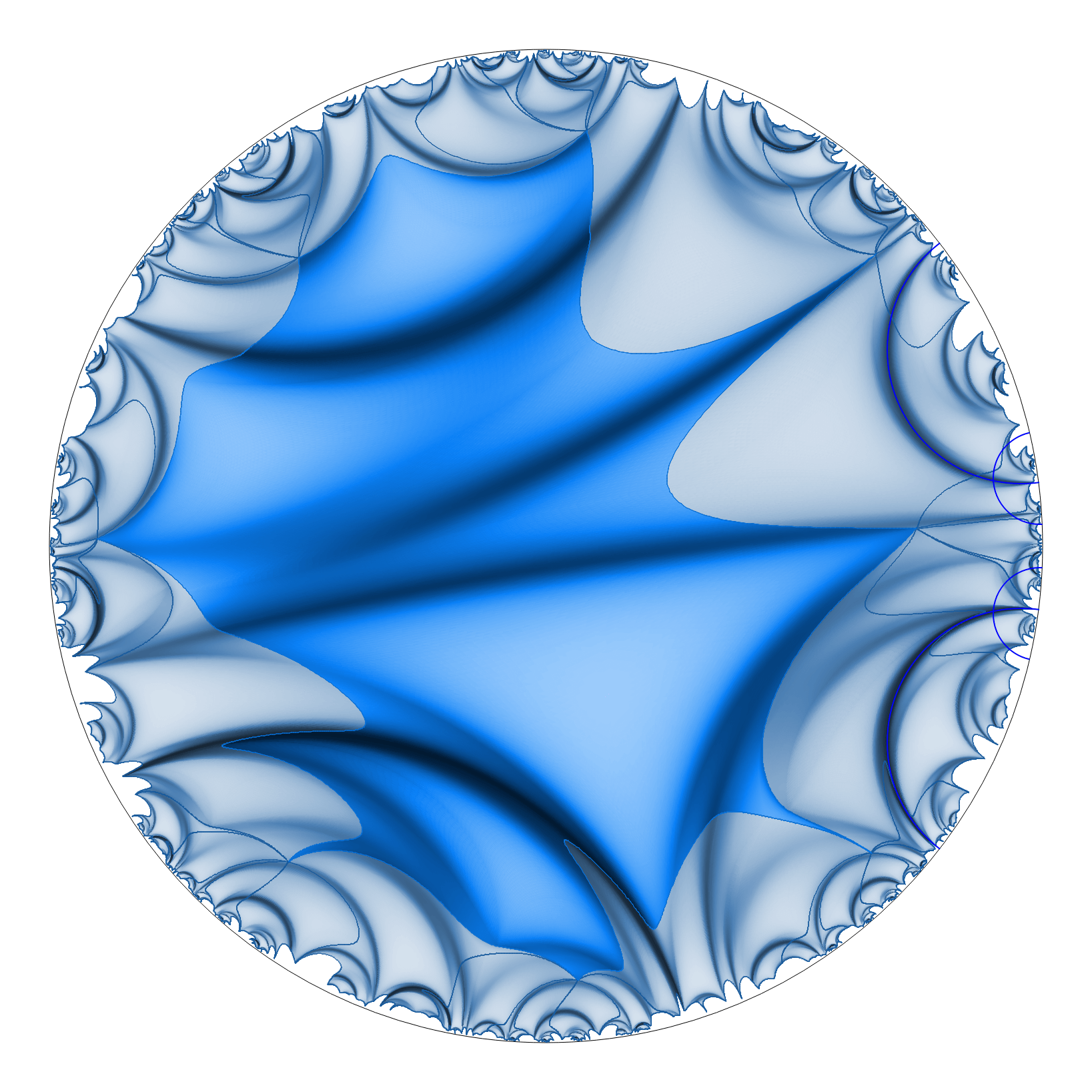
Geometric structures on low-dimensional manifolds, surface
Most compact surfaces without boundary can be equipped with hyperbolic metrics, or, equivalently, with complex structures. The space of all those structures, called the Teichmüller space of the surface, has a rich geometry. One way to understand this geometry is through closely related geometric structures on 3-dimensional manifolds: quasifuchsian hyperbolic structures, or their Lorentzian cousin
Head of Group
