Topics In High-dimensional Stochastic Analysis (HDSA)

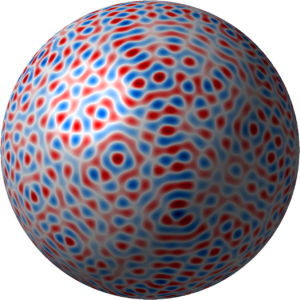
Probability theory is the branch of mathematics dealing with the rigorous study of random phenomena. The aim of this project is to study new ways of quantifying and controlling the random fluctuations of large random systems, emerging in several domains, such as Statistics, Mathematical Physics and Stochastic Geometry. One of the themes common to the many venues explored in the project is that of applying variational techniques, involving several stochastic (and possibly, infinite-dimensional) versions of derivative and difference operators, as well as integration by parts formulae, from classical analysis. The aim of this project is to develop new probabilistic and analytical techniques, in order to explore several open venues for research in the broad area of stochastic analysis, with specific emphasis on the following domains: quantitative multidimensional limit theorems, quantitative functional approximations, random geometric graphs, variance estimates, phase transitions and noise sensitivity, percolative properties of continuum models, the geometry of excursion sets of random fields. The crucial theme overarching the proposed research is the use of tools from stochastic calculus, and in particular, tools related to the so-called “Malliavin calculus of variations”, that we plan to apply both in Gaussian and discrete settings. The planned research is subdivided into three interconnected parts, that we have labelled A, B and C. In Part A, we propose (A-1) to create an efficient theory of multidimensional quantitative weak and strong stabilization for vectors of random variables that depend on a Gaussian input, and to develop a number of geometric and non-geometric applications, (A-2) to develop quantitative bounds for Poisson-based spatial central limit theorems, with special attention to models of combinatorial optimization and to the fundamental notion of multivariate weak geometric stabilization, (A-3) to attack the open problem of quantifying the rates of convergence in functional limit theorems involving random element taking values in metric space (like the Skorohod space of cadlag mappings), and (A-4) to initiate a systematic study of the functional convergence of empirical processes based on random geometric graphs. Part B will focus on the following tasks: (B1) to develop a collection of new results, related to the variational analysis of random variables on configuration spaces depending on stopping sets, with the special aim of extending and unifying the proofs of the recently established intrinsic versions of the OSSS and Schramm-Steif inequalities on configuration spaces, (B2) to use and generalize the two inequalities evoked above in order to study sharp phase transitions and noise sensitivity of several models of continuum percolation, (B3) to initiate the study of “continuum spectral samples” associated with functionals of a Poisson measure, and (B4) to provide a complete study of the “restricted hypercontractivity” phenomenon on the Poisson space, with applications to generalized Talagrand-type inequalities, through the possible use of techniques of continuous-time stochastic calculus. Finally, Part C will revolve around the following goals: (C1) to develop a global theory of fluctuations for local geometric quantities associated with random fields on manifolds, by using tools of Gaussian analysis as well as coupling and randomization devices, one fundamental aim being the characterization of the global fluctuations of nodal volumes associated with monochromatic random waves on general 2-manifolds, (C2) to provide a systematic study of local geometric quantities associated with non-Gaussian random waves, and in particular with non-Gaussian random waves generated by random point measures, (C3) to initiate a systematic study of functional fluctuations for geometric empirical processes related to random fields on manifolds. The content of part (C1) also relates to important general questions about the efficient coupling of Gaussian random elements in Banach spaces. The scientific activities associated with the project will require the hiring of two post-doctoral fellows, each joining the PI’s research group for a period of two years. The proposal also involves the organization of a scientific conference about Stochastic Analysis to be held during the third year.