Research at the Quantum Theory Group
Our group investigates non-equilibrium quantum systems with applications to Quantum Science and Technology and Foundations of Physics.
Quantum Control – Shortcuts to Adiabaticity
Shortcuts to adiabaticity have been recently been proposed as a general tool to tailor the nonadiabatic dynamics of quantum matter far from equilibrium. They provide control protocols to guide the dynamics of a quantum system through an adiabatic reference trajectory in an arbitrary prescheduled time, without the requirement of slow driving. As an alternative to adiabatic protocols, they have found broad applications in quantum science and technology. We have recently generalized these techniques to open quantum systems.
Adiabatic Quantum Computation
Bringing ideas of critical, quantum open systems and nonequilibrium phenomena we have advanced heuristic protocols and algorithms for quantum annealing. These include the introduction of shortcuts to adiabaticity related to quantum gatchets, spatially inhomogeneous annealing schedules that have recently gained in popularity, and the choice of optimal annealing times in the presence of noise. We have also proposed the benchmarking of quantum annealers by testing principles of nonequilibrium statistical mechanics such as the defect distribution as a function of the annealing time, supporting the fact that current D-Wave quantum annealers are best described as open quantum systems.
Dynamics of Phase transitions and Topological Defects
Symmetry breaking phase transitions play an important role in Nature. When a system traverses a transition of this type at a finite rate, its causally disconnected regions choose the new broken-symmetry state independently. Where such local choices are incompatible, defects form with densities that are predicted to follow a power law scaling in the rate of the transition. The importance of this universal Kibble-Zurek mechanism ranges from cosmology to condensed matter. This mechanism is expected to limit the performance of Quantum Annealers which solve complex optimization problems by encoding the solution of interest in the ground-state of a quantum physical system.
Quantum Speed Limits and Information Geometry
The multi-faceted nature of time makes its treatment challenging in the quantum world. Nonetheless, the understanding of time-energy uncertainty relations is somewhat privileged. To a great extent, this is due to their reformulation in terms of quantum speed limits (QSL), concerning the ability to distinguish two quantum states connected via time evolution. While QSL provide fundamental constraints to the pace at which quantum systems can change, a plethora of applications have been found that well extend beyond the realm of quantum dynamics. Indeed, QSL provide limits to the computational capability of physical devices, the performance of quantum thermal machines in finite-time thermodynamics, parameter estimation in quantum metrology, quantum control, the decay of unstable quantum systems and information scrambling, among other examples.
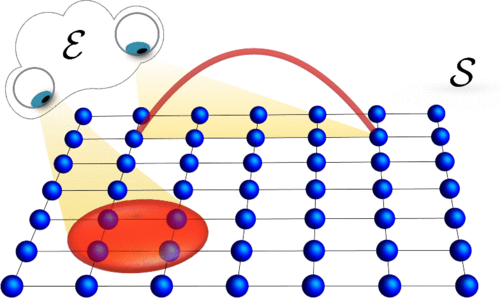
Open and Noisy Quantum Systems
Any physical system is embedded in an environment. As a result of this coupling the system is said to be open, and its dynamics is often governed by decoherence. We study the dynamics of open systems with an eye on quantum technologies including adiabatic quantum annealing and quantum metrology.
In addition, we explore quantum open systems in relation to foundations of physics, e.g. the role of time in quantum mechanics.
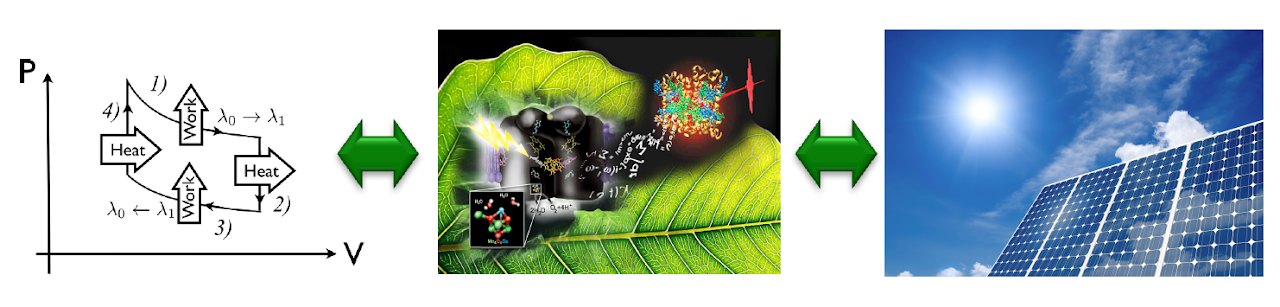
Quantum Thermodynamics
The interplay of quantum technology and foundations of physics has turned quantum thermodynamics into a blooming field. With the miniaturization to the nanoscale, a need has emerged to understand and control the dynamics of devices operating in the presence of thermal and quantum fluctuations. Quantum heat engines (QHEs) constitute a prominent example, targeting the efficient conversion of heat into mechanical work. The relevance of this goal is further strengthened by the connection between QHEs and both natural and artificial light harvesting systems as well as by the progress in the experimental realization of heat engines and refrigerators at the nanoscale.
Strongly coupled quantum systems – in and out of
equilibrium
The understanding of strongly-coupled quantum systems is an open problem at the frontiers of physics with widespread applications. Prominent examples include the description of atomic nuclei, quark-gluon plasma, quantum liquids and other exotic phases of matter. Understanding systems made of strongly-interacting particles is generally a challenging task as most of the theoretical tools at hand (e.g., perturbation theory, notion of quasi-particles, etc.) fail. In ultracold atom physics, a unitary Fermi gas constitutes an excellent test-bed for physics at strong coupling.
Other experimental prospects are at reach in the field of quantum simulation.